

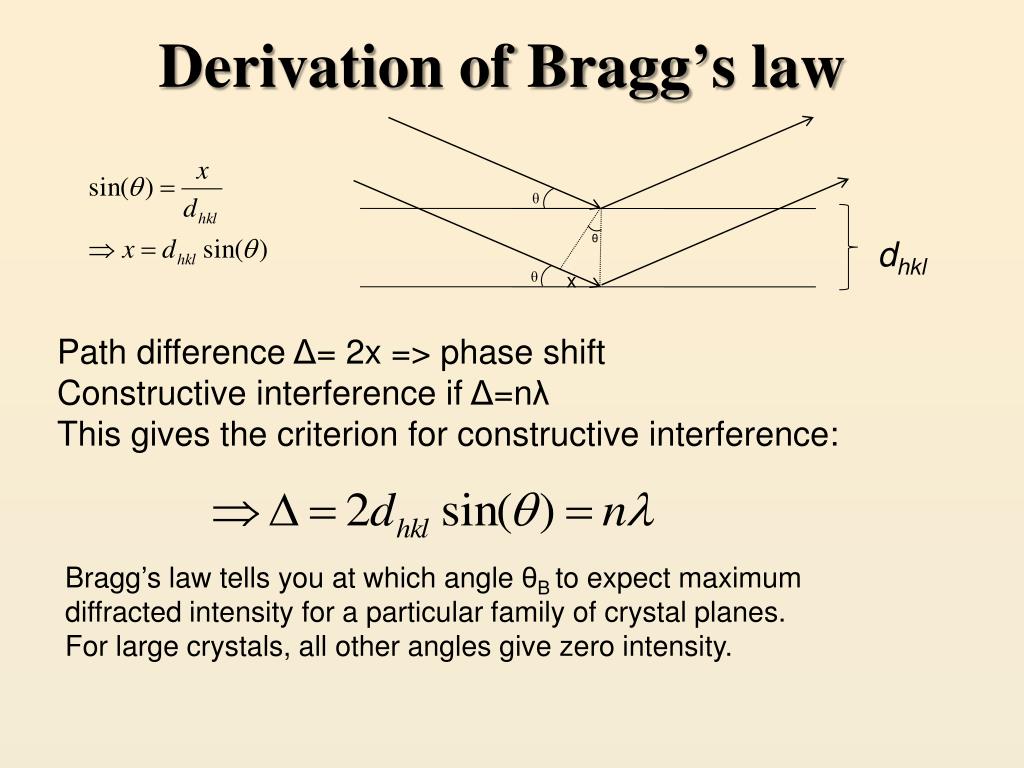
As a result of the diffraction limit, two emitting points cannot be optically resolved if the distance between them is smaller than the diffraction limit, which is illustrated in figure 1 (b). In practical applications, this difference is small. In a perfect optical system without any aberrations, the PSF is well-described by the so called Airy function. The difference between the two is based on the definition that both Abbe and Rayleigh used in their derivation for what is meant by two objects being resolvable from each other. The PSF is the response of an optical system to a point emitter due to the diffraction limit and imperfections in the optical system. As in Fresnel diffraction, we’ll typically assume a plane wave incident field, we’ll neglect the phase factors, and we’ll explicitly write the aperture function in the integral: 00 01 01 1 1 1 1,exp (,) jk E x y x x y y Aperture x y dx dy z Fraunhofer Diffraction: when the quadratic terms can be ignored. In more mathematical sense it can also be said that the resulting image is a convolution of the actual object with the so-called point spread function (PSF) of the optical system. This results in a blurry appearance of the captured image. The high frequency components that give an image its sharpness are lost by the finite numerical aperture of the lens that collects the light. The limit is basically a result of diffraction processes and the wave nature of light. The numerical aperture (NA) and the resolution limit is schematically illustrated in figure 1. According to the derivation of Fresnel diffraction, successful reconstructions can only be obtained when the small-angle number, a derived quantity, is much. Only more recently have scientists revisited Abbe's limit and successfully increased the resolution of light microscopy through a variety of innovative strategies (see Milestone 21).D = \frac The diffraction-limited resolution theory was advanced by German physicist Ernst Abbe in 1873 (see Equation (1)) and later refined by Lord Rayleigh in 1896 ( Equation (3)) to quantitate the measure of separation necessary between two Airy patterns in order to distinguish them as separate entities. The principal reason limiting the resolution is the diffraction of light waves. capability to generate distinguishable images of close objects. Can’t seem to find a derivation of it on the web optics diffraction microscopy Share. Rather, other techniques were developed to provide these data, such as replacing photons with electrons in the development of electron microscopy. Abbe’s theory Any imaging system has a finite limit of resolution, i.e. From my research I came across many interesting concepts like Huygen’s Principle which completely changed my view on diffraction and Abbe’s limit which is. The first minimum is at an angle of 1.22 / D, so that two point objects are just resolvable. The beam spreads out with an angle given by the equation. The Rayleigh criterion for the diffraction limit to resolution states that two images are just resolvable when the center of the diffraction pattern of one is directly over the first minimum of the diffraction pattern of the other. In fact, Abbe's study influenced the field so greatly that very few attempts were made to overcome the diffraction limit, despite the increasing necessity to enhance resolution and to improve the visualization of cellular structure. When the observer stays in the near field, the Abbe’s theory on the diffraction limit ( Abbe, 1873) also known as Rayleigh criterion is no longer applicable and the resolution is only determined by the size of the light source and its separation from the surface. The Rayleigh criterion is given as: m i n 1.22 D. Any beam of light having a finite diameter D and a wavelength exhibits diffraction spreading. But besides these tangible legacies, another long-lasting legacy brought about by Abbe's work included the establishment of physical boundaries in imaging for quite some time. The first minimum is at an angle of 1.22/ D, so that two point objects are just resolvable.
Of course, the success of Abbe, Zeiss and Schott in designing lenses also had an enormous impact on the eventual success of the microscopy manufacturer Carl Zeiss itself. The Rayleigh criterion for the diffraction limit to resolution states that two images are just resolvable when the centre of the diffraction pattern of one is directly over the first minimum of the diffraction pattern of the other. Abbe's quantitative insights greatly enhanced the quality of microscope optics, contributing enormously to improved data collection and an enhanced user experience for the microscopist.
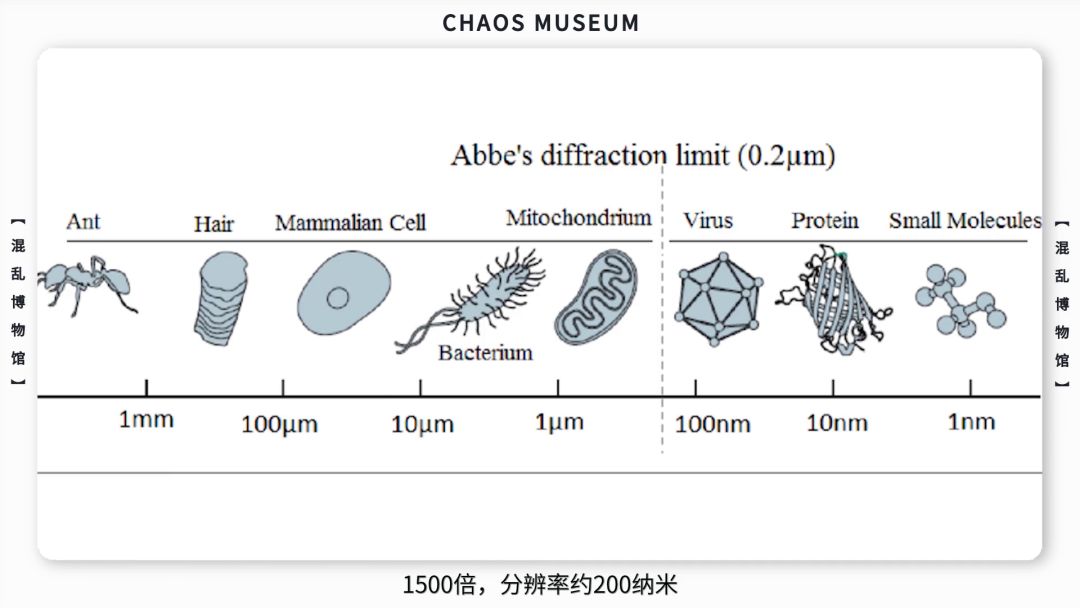
Abbe's mathematical foundations of image formation and lens aberrations provided for the proper design of microscope lenses, accomplished in collaboration with Carl Zeiss and Otto Schott. In case the resolution is assumed to be limited only by wave diffraction, Abbes resolution limit is the key figure 3.


 0 kommentar(er)
0 kommentar(er)
